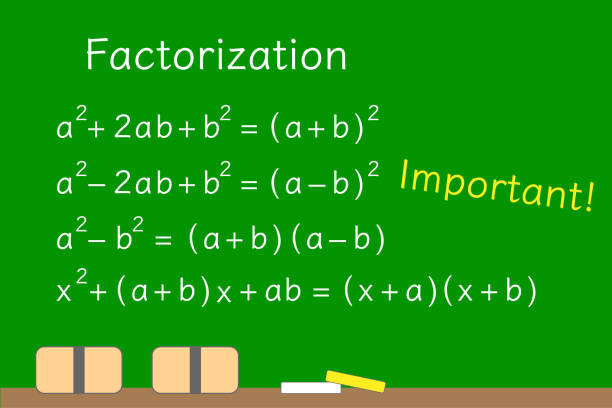
Prime factorization is a way of expressing a number as a product of its prime factors. A prime number is a number that has exactly two factors, 1 and the number itself. For example, the number 5 is a prime number because its only factors are 1 and 5. The number 18 is composite because it has factors other than 1 and 18, such as 2, 3, 6, and 9.
One of the most basic operations in mathematics is the prime factorization of a number. For example, the number 21 is composed of the prime numbers 3 and 7. However, what if we want to find the set of all the prime numbers less than a certain value? For example, what is the prime factorization of 6? The answer is pretty simple, 3 and 2.
Factorization is a way of reducing a composite number to its basic elements. In our number example, 2 can be factored into its prime factors, 2 and 1. In this article, we’ll focus on prime factorization, which is the division of a number into only its prime factors. In other words, a number will be divisible by only one of its prime factors.
Here is a list of the most commonly used methods to prime factor a number:
- Prime factorization using factor tree method– The factor tree method is one of the most basic and commonly used methods to prime factorize a number. In this method, the factorization of a number is represented as a tree where each node in the tree represents a prime factor. The leaves of the tree represent the prime numbers and the path between two leaves represents a factorization of the number. For example, the factorization of 6 can be represented as 3*2, which can be read as “three goes into two twice”.
- Prime factorization using division method- The division method is one of the most basic and commonly used methods to prime factorize a number. In this method, the factorization of a number is represented as a division problem. The dividend is the number to be factored and the divisor is the number to be found through the factorization of the number. The factorization of 6 can be represented as 3 dividable by 2, which can be read as “three is a divisor of 2”.
Applications of the prime factorization are very diverse, and it’s often used in practical applications. One of the most common uses of prime factorization is finding the prime factors of a given number. Moreover, in an Elliptic Curve Cryptography (ECC) implementation, prime numbers are used to generate a private key. In an RSA implementation, prime numbers are used to generate a public and a private key. Thus, below are some of the applications of prime factorization.
- Cryptography- Cryptography is the study of methods of securing the information so that only authorized individuals can read it. Cryptography is used in many applications, such as banking, e-commerce, and data storage. In cryptography, prime numbers are used to generate a private key. In an RSA implementation, prime numbers are used to generate a public and a private key.
- HCF and LCM- The factorization of a number is an important step in finding its prime factors. One of the most basic and commonly used methods to prime factorize a number is the HCF and LCM methods. The HCF and LCM methods are two of the most basic factorization methods. The HCF and LCM methods are used to reduce a composite number to its prime factors.
Thus, prime factorization is an important concept to learn. It has many applications in the future. If you want to understand this concept better then, Cuemath is the place to go. They have the best resources on topics like factors, prime factorization, greatest common factor, HCF, LCM, etc.